foryoulobi.blogg.se

Another common use can be found in medicine in endoscopes. Optical fibers are commonly used in telecommunications, because information can be transported over long distances, with minimal loss of data. In this way, light travels very quickly down the length of the cable over a very long distance (tens of kilometers). If light is incident on a cable end with an angle of incidence greater than the critical angle then the light will remain trapped inside the glass strand. The difference in refractive index of the cladding and the core allows total internal reflection in the same way as happens at an air-water surface show in. The overall diameter of the fiber is about 125 μm and that of the core is just about 50 μm. The basic functional structure of an optical fiber consists of an outer protective cladding and an inner core through which light pulses travel. This shows a single fiber with its cladding. The construction of a single optical fibre is shown in.įig 2: Fibers in bundles are clad by a material that has a lower index of refraction than the core to ensure total internal reflection, even when fibers are in contact with one another. An optical fibre is a thin, transparent fibre, usually made of glass or plastic, for transmitting light. One of the most common applications of total internal reflection is in fibre optics. Total internal reflection is a powerful tool since it can be used to confine light. So the critical angle is only defined when n 2/n 1 is less than 1.įig 1: Refraction of light at the interface between two media, including total internal reflection. The resulting value of θ1θ1 is equal to the critical angle θc=θ1=arcsin(n2n1)θc=θ1=arcsin⁡(n2n1). To find the critical angle, we find the value for θ1θ1 when θ2θ2= 90° and thus sinθ2=1sin⁡θ2=1. Here, n 1 and n 2 are refractive indices of the media, and θ1θ1 and θ2θ2are angles of incidence and refraction, respectively.

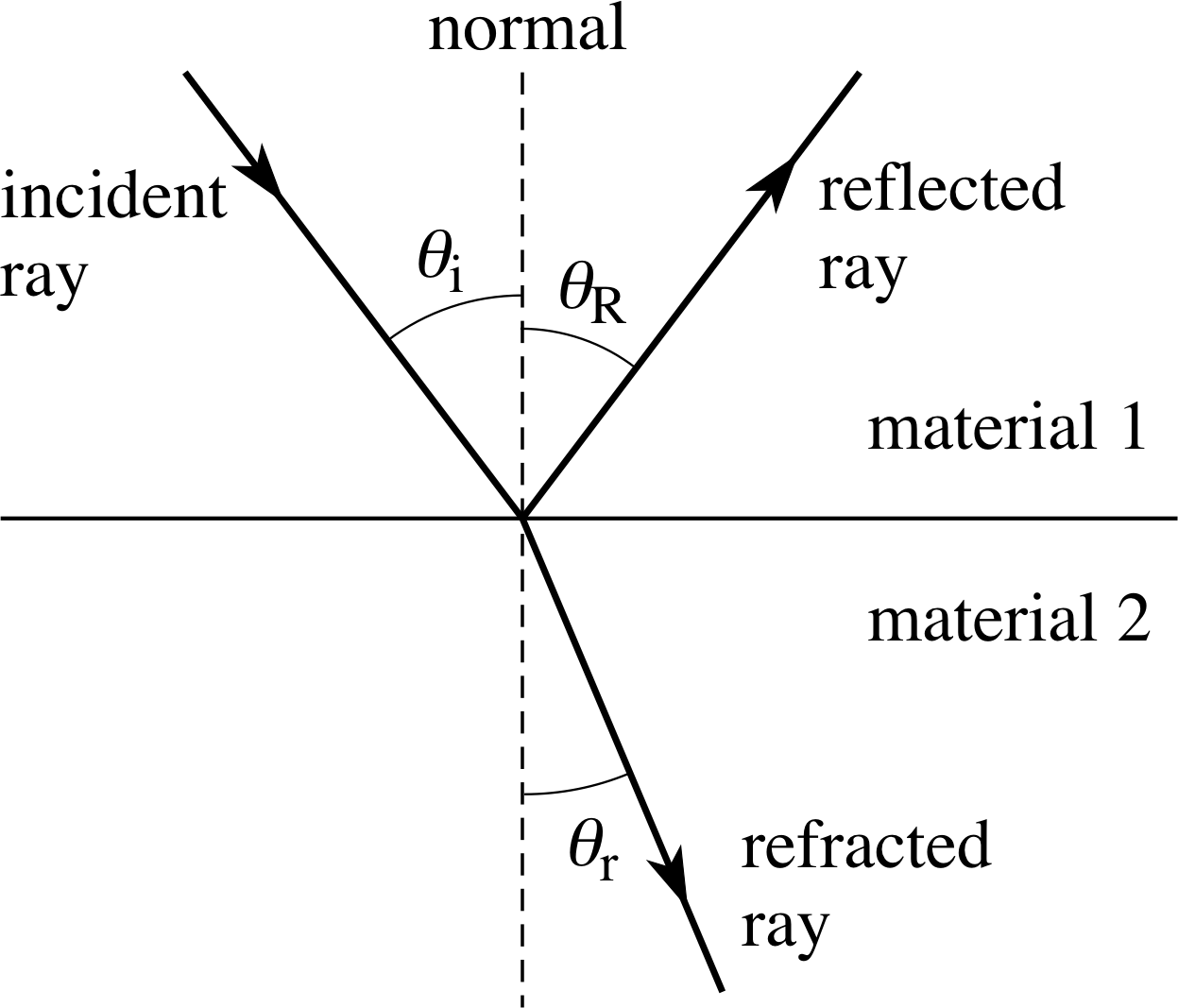
The critical angle θcθc is given by Snell’s law, n1sinθ1=n2sinθ2n1sin⁡θ1=n2sin⁡θ2. It is at this point no light is transmitted into air. When the incident angle is increased sufficiently, the transmitted angle (in air) reaches 90 degrees. The light emanating from the interface is bent towards the glass. Consider a light ray passing from glass into air. The angle of incidence is measured with respect to the normal at the refractive boundary (see diagram illustrating Snell’s law ). The critical angle is the angle of incidence above which total internal reflection occurs. Understanding Snell’s Law with the Index of Refraction: This video introduces refraction with Snell’s Law and the index of refraction.The second video discusses total internal reflection (TIR) in detail. Snell’s experiments showed that the law of refraction was obeyed and that a characteristic index of refraction n could be assigned to a given medium. The law of refraction is also called Snell’s law after the Dutch mathematician Willebrord Snell, who discovered it in 1621. The incoming ray is called the incident ray and the outgoing ray the refracted ray, and the associated angles the incident angle and the refracted angle.

Here n 1 and n 2 are the indices of refraction for medium 1 and 2, and θ 1 and θ 2are the angles between the rays and the perpendicular in medium 1 and 2.